Eureka Math Grade 4 Module 5 Lesson 16 Answer Key (2024)

Engage NY Eureka Math 4th Grade Module 5 Lesson 16 Answer Key

Eureka Math Grade 4 Module 5 Lesson 16 Problem Set Answer Key

Question 1.
Solve.
a. 3 fifths – 1 fifth = _____2 __fifths_____

Answer:
3 fifths – 1 fifth = 2 fifths.

Explanation:
In the above-given question,
given that,
3 fifths = 3/5.
3/5 = 0.6.
1 fifth = 1/5.
1/5 = 0.2.
0.6 – 0.2 = 0.4.
2/5 = 0.4.
3 fifths – 1 fifth = 2 fifths.

b. 5 fifths – 3 fifths = _____2_fifths._________

Answer:
5 fifths – 3 fifth = 2 fifths.

Explanation:
In the above-given question,
given that,
5 fifths = 5/5.
5/5 = 1.
3 fifth = 3/5.
3/5 = 0.6.
1 – 0.6 = 0.4.
2/5 = 0.4.
3 fifths – 1 fifth = 2 fifths.

c. 3 halves – 2 halves = _____1_ halves._____

Answer:
3 halves – 2 halves = 1 halves

Explanation:
In the above-given question,
given that,
3 halves = 3/2.
3/2 = 1.5.
2 halves = 2/2.
2/2 = 1.
1.5 – 1 = 0.5.
1/2 = 0.5.
3 halves – 2 halves = 1 half.

d. 6 fourths – 3 fourths = ____3__fourths._______

Answer:
6 fourths – 3 fourths = 3 fourths.

Explanation:
In the above-given question,
given that,
6 fourths = 6/4.
6/4 = 1.5.
3 fourths = 3/4.
3/4 = 0.75.
1.5 – 0.75 = 0.75.
3/4 = 0.75.
6 fourths – 3 fourths = 3 fourths.

Question 2.
Solve.

a. \(\frac{5}{6}\) – \(\frac{3}{6}\)

Answer:
\(\frac{5}{6}\) – \(\frac{3}{6}\) = \(\frac{2}{6}\)

Explanation:
In the above-given question,
given that,
5 sixths = 5/6.
5/6 = 0.83.
3 sixths = 3/6.
3/6 = 0.5.
0.83 – 0.5 = 0.33.
2/6 = 0.33.
\(\frac{5}{6}\) – \(\frac{3}{6}\) = \(\frac{2}{6}\)

b. \(\frac{6}{8}\) – \(\frac{4}{8}\)

Answer:
\(\frac{6}{8}\) – \(\frac{4}{8}\) = \(\frac{2}{8}\)

Explanation:
In the above-given question,
given that,
6 eights = 6/8.
6/8 = 0.75.
4 eights = 4/8.
4/8 = 0.5.
0.75 – 0.5 = 0.25.
2/8 = 0.25.
\(\frac{6}{8}\) – \(\frac{4}{8}\) = \(\frac{2}{8}\)

c. \(\frac{3}{10}\) – \(\frac{3}{10}\)

Answer:
\(\frac{3}{10}\) – \(\frac{3}{10}\) = \(\frac{0}{0}\)

Explanation:
In the above-given question,
given that,
3 tens = 3/10.
3/10 = 0.3.
3 tens = 3/10.
3/10 = 0.3.
0.3 – 0.3 = 0.
0/6 = 0.
\(\frac{3}{10}\) – \(\frac{3}{10}\) = \(\frac{0}{0}\)

d. \(\frac{5}{5}\) – \(\frac{4}{5}\)

Answer:
\(\frac{5}{5}\) – \(\frac{4}{5}\) = \(\frac{1}{5}\)

Explanation:
In the above-given question,
given that,
5 fifths = 5/5.
5/5 = 1.
4 fifths = 4/5.
4/5 = 0.8.
1 – 0.8 = 0.2.
1/5 = 0.2.
\(\frac{5}{5}\) – \(\frac{4}{5}\) = \(\frac{1}{5}\)

e. \(\frac{5}{4}\) – \(\frac{4}{4}\)

Answer:
\(\frac{5}{4}\) – \(\frac{4}{4}\) = \(\frac{1}{4}\)

Explanation:
In the above-given question,
given that,
5 fours = 5/4.
5/4 = 1.25.
4 fours = 4/4.
4/4 = 1.
1.25 – 1 = 0.25.
1/4 = 0.25.
\(\frac{5}{4}\) – \(\frac{4}{4}\) = \(\frac{1}{4}\)

f. \(\frac{5}{4}\) – \(\frac{3}{4}\)

Answer:
\(\frac{5}{4}\) – \(\frac{3}{4}\) = \(\frac{2}{4}\)

Explanation:
In the above-given question,
given that,
5 fours = 5/4.
5/4 = 1.25.
3 fours = 3/4.
3/4 = 0.75.
1.25 – 0.75 = 0.5.
2/4 = 0.5.
\(\frac{5}{4}\) – \(\frac{3}{4}\) = \(\frac{2}{4}\)

Question 3.
Solve. Use a number bond to show how to convert the difference to a mixed number. Problem (a) has been completed for you.
a.

Answer:
12/8 – 3/8 = 9/8.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
12/8 – 3/8.
9/8.
9/8 = 8/8 + 1/8.

b. \(\frac{12}{6}\) – \(\frac{5}{6}\)

Answer:
12/6 – 5/6 = 7/6.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
12/6 – 5/6.
7/6.
7/6 = 6/6 + 1/6.

c. \(\frac{9}{5}\) – \(\frac{3}{5}\)

Answer:
9/5 – 3/5 = 6/5.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
9/5 – 3/5.
6/5.
6/5 = 5/5 + 1/5.

d. \(\frac{14}{8}\) – \(\frac{3}{8}\)

Answer:
14/8 – 3/8 = 11/8.

Eureka Math Grade 4 Module 5 Lesson 16 Exit Ticket Answer Key

Question 1.
Solve. Use a number bond to decompose the difference. Record your final answer as a mixed number.
\(\frac{16}{9}\) – \(\frac{5}{9}\)

Answer:
16/9 – 5/9 = 11/9.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
16/9 – 5/9.
11/9.
11/9 = 9/9 + 2/9.

Question 2.
Solve. Use a number bond to decompose the sum. Record your final answer as a mixed number.
\(\frac{5}{12}\) + \(\frac{10}{12}\)

Answer:
5/12 + 10/12 = 15/12.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
5/12 + 10/12.
15/12.
15/12 = 12/12 + 3/12.

Eureka Math Grade 4 Module 5 Lesson 16 Homework Answer Key

Question 1.
Solve.
a. 3 sixths – 2 sixths = __1__sixths___________

Answer:
3 sixths – 2 sixths = 1 sixths.

Explanation:
In the above-given question,
given that,
3 sixths = 3/6.
3/6 = 0.5.
2 sixths = 2/6.
2/6 = 0.33.
0.5 – 0.3 = 0.1.
1/6 = 0.1.

b. 5 tenths – 3 tenths = ___2 tenths._____

Answer:
5 tenths – 3 tenths = 2 tenths.

Explanation:
In the above-given question,
given that,
5 tenths = 5/10.
5/10 = 0.5.
3 tenths = 3/10.
3/10 = 0.3.
0.5 – 0.3 = 0.2.
2/10 = 0.2.

c. 3 fourths – 2 fourths = ____1__fourth._________

Answer:
3 fourths – 2 fourths = 1 fourth.

Explanation:
In the above-given question,
given that,
3 fourths = 3/4.
3/4 = 0.75.
2 fourths = 2/4.
2/4 = 0.5.
0.75 – 0.5 = 0.25.
1/4 = 0.25.

d. 5 thirds – 2 thirds = ___3__thirds._____

Answer:
5 thirds – 2 thirds = 3 thirds.

Explanation:
In the above-given question,
given that,
5 thirds = 5/3.
3/5 = 1.6.
2 thirds = 2/3.
2/3 = 0.6.
1.6 – 0.6 = 1.
3/3 = 1.

Question 2.
Solve.

a. \(\frac{3}{5}\) – \(\frac{2}{5}\)

Answer:
\(\frac{3}{5}\) – \(\frac{2}{5}\) = \(\frac{1}{5}\)

Explanation:
In the above-given question,
given that,
3 fives = 3/5.
3/5 = 0.6.
2 fives = 2/5.
2/5 = 0.4.
0.6 – 0.4 = 0.2.
1/5 = 0.2.
\(\frac{3}{5}\) – \(\frac{2}{5}\) = \(\frac{1}{5}\)

b. \(\frac{7}{9}\) – \(\frac{3}{9}\)

Answer:
\(\frac{7}{9}\) – \(\frac{3}{9}\) = \(\frac{4}{9}\)

Explanation:
In the above-given question,
given that,
7 nines = 7/9.
7/9 = 0.7.
3 nines = 3/9.
3/9 = 0.3.
0.7 – 0.3 = 0.4.
4/9 = 0.4.
\(\frac{7}{9}\) – \(\frac{3}{9}\) = \(\frac{4}{9}\)

c. \(\frac{7}{12}\) – \(\frac{3}{12}\)

Answer:
\(\frac{7}{12}\) – \(\frac{3}{12}\) = \(\frac{4}{12}\)

Explanation:
In the above-given question,
given that,
7 twelfths = 7/12.
7/12 = 0.58.
3 twelfths = 3/12.
3/12 = 0.25.
0.58 – 0.25 = 0.33.
4/12 = 0.33.
\(\frac{7}{12}\) – \(\frac{3}{12}\) = \(\frac{4}{12}\)

d. \(\frac{6}{6}\) – \(\frac{4}{6}\)

Answer:
\(\frac{6}{6}\) – \(\frac{4}{6}\) = \(\frac{2}{6}\)

Explanation:
In the above-given question,
given that,
6 sixs = 6/6.
6/6 = 1.
4 sixs = 4/6.
4/6 = 0.6.
1 – 0.6 = 0.4.
2/6 = 0.4.
\(\frac{6}{6}\) – \(\frac{4}{6}\) = \(\frac{2}{6}\)

e. \(\frac{5}{3}\) – \(\frac{2}{3}\)

Answer:
\(\frac{5}{3}\) – \(\frac{2}{3}\) = \(\frac{3}{3}\)

Explanation:
In the above-given question,
given that,
5 threes = 5/3.
5/3 = 1.6.
2 threes = 2/3.
2/3 = 0.6.
1.25 – 1 = 0.25.
3/3 = 1.
\(\frac{5}{3}\) – \(\frac{2}{3}\) = \(\frac{3}{3}\)

f. \(\frac{7}{4}\) – \(\frac{5}{4}\)

Answer:
\(\frac{7}{4}\) – \(\frac{5}{4}\) = \(\frac{3}{4}\)

Explanation:
In the above-given question,
given that,
7 fours = 7/4.
7/4 = 1.75.
5 fours = 4/4.
5/4 = 1.25.
1.75 – 1.25 = 0.75.
3/4 = 0.75.
\(\frac{7}{4}\) – \(\frac{5}{4}\) = \(\frac{3}{4}\)

Question 3.
Solve. Use a number bond to decompose the difference. Record your final answer as a mixed number. Problem (a) has been completed for you.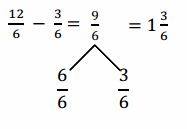

Answer:
12/6 – 3/6 = 9/6.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
12/6 – 3/4.
9/6.
9/6 = 6/6 + 3/6.

b. \(\frac{17}{8}\) – \(\frac{6}{8}\)

Answer:
17/8 – 6/8 = 11/8.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
17/8 – 6/8.
11/8.
11/8 = 8/8 + 2/8.

c. \(\frac{9}{5}\) – \(\frac{3}{5}\)

Answer:
9/5 – 3/5 = 6/5.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
9/5 – 3/5.
6/5.
6/5 = 5/5 + 1/6.

d. \(\frac{11}{4}\) – \(\frac{6}{4}\)

Answer:
11/4 – 6/4 = 5/4.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
11/4 – 6/4.
5/4.
5/4 = 4/4 + 1/4.

e. \(\frac{10}{7}\) – \(\frac{2}{7}\)

Answer:
10/7 – 2/7 = 8/7.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
10/7 – 2/7.
8/7.
8/7 = 7/7 + 1/7.

f. \(\frac{21}{10}\) – \(\frac{9}{10}\)

Answer:
21/10 – 9/10 = 11/10.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
21/10 – 9/10.
11/10.
11/10 = 10/10 + 1/10.

Question 4.
Solve. Write the sum in unit form.
a. 4 fifths + 2 fifths = ____6__fifths.____

Answer:
4 fifths + 2 fifths = 6 fifths.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
4 fifths = 1/5 + 1/5 + 1/5 + 1/5.
2 fifths = 1/5 + 1/5.
4 fifths + 2 fifth = 6 fifths.
1/5 + 1/5 + 1/5 + 1/5 + 1/5 + 1/5 = 6/5.

b. 5 eighths + 2 eighths = __7___eights.______

Answer:
5 eights + 2 eighths = 7 eights.

Explanation:
In the above-given question,
given that,
Use a number bond to show how to convert the difference to a mixed number.
5 eights = 1/8 + 1/8 + 1/8 + 1/8 + 1/8.
2 eights = 1/8 + 1/8.
5 eights + 2 eights = 7 eights.
1/8 + 1/8 + 1/8 + 1/8 + 1/8 + 1/8 + 1/8 = 7/8.

Question 5.
Solve.
a. \(\frac{3}{11}\) + \(\frac{6}{11}\)

Answer:
3/11 + 6/11 = 9/11.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
3/11 + 6/11.
9/11.
9/11 = 3/11 + 9/11.

b. \(\frac{3}{10}\) + \(\frac{6}{10}\)

Answer:
3/10 + 6/10 = 9/10.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
3/10 + 6/10.
9/10.
9/10 = 3/10 + 6/10.

Question 6.
Solve. Use a number bond to decompose the sum. Record your final answer as a mixed number.
a. \(\frac{3}{4}\) + \(\frac{3}{4}\)

Answer:
3/4 + 3/4 = 6/4.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
3/4 + 3/4.
6/4.
6/4 = 4/4 + 5/4.

b. \(\frac{8}{12}\) + \(\frac{6}{12}\)

Answer:
8/12 + 6/12 = 14/12.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
8/12 + 6/12.
14/12.
14/12 = 12/12 + 2/12.

c. \(\frac{5}{8}\) + \(\frac{7}{8}\)

Answer:
5/8 + 7/8 = 12/8.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
5/8 + 7/8.
12/8.
12/8 = 8/8 + 4/8.

d. \(\frac{8}{10}\) + \(\frac{5}{10}\)

Answer:
8/10 + 5/10 = 13/10.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
8/10 + 5/10.
13/10.
13/10 = 10/10 + 3/10.

e. \(\frac{3}{5}\) + \(\frac{6}{5}\)

Answer:
3/5 + 6/5 = 9/5.

Explanation:
In the above-given question,
given that,
Use a number bond to decompose the sum.
3/5 + 6/5.
9/5.
9/5 = 5/5 + 4/5.

Question 7.
Solve. Use a number line to model your answer.

a. \(\frac{11}{9}\) – \(\frac{5}{9}\)

Answer:
\(\frac{11}{9}\) – \(\frac{5}{9}\) = \(\frac{6}{9}\)

Explanation:
In the above-given question,
given that,
11 nines = 11/9.
11/9 = 0.58.
5 nines = 5/9.
5/9 = 0.25.
0.58 – 0.25 = 0.33.
6/9 = 0.33.
\(\frac{11}{9}\) – \(\frac{5}{9}\) = \(\frac{6}{1}\)

b. \(\frac{13}{12}\) + \(\frac{4}{12}\)

Eureka Math Grade 4 Module 5 Lesson 16 Answer Key (2024)

FAQs

What grade does Eureka math go up to? ›

Eureka Math^® is a holistic Prekindergarten through Grade 12 curriculum that carefully sequences mathematical progressions in expertly crafted modules, making math a joy to teach and learn. We provide in-depth professional development, learning materials, and a community of support.

Know More ›

What are the four core components of a Eureka Math TEKS lesson? ›

A typical Eureka lesson is comprised of four critical components: fluency practice, concept development (including a problem set), application problem, and student debrief (including the Exit Ticket).

What is the purpose of the concept development in Eureka math? ›

The concept development is generally comprised of carefully sequenced problems centered within a specific topic to begin developing mastery via gradual increases in complexity.

See Details ›

What is the hardest math grade? ›

The hardest math class you can take in high school is typically AP Calculus BC or IB Math HL. These courses cover a wide range of advanced mathematical concepts, including calculus, trigonometry, and statistics.

Learn More ›

Is Eureka Math good or bad? ›

Is Eureka Math a good curriculum? The answer to this question depends on the target audience. If you're a teacher in a public school who needs to cover State Standards and your goal is merely to prepare students for State tests, then Eureka may be a good curriculum for you.

Show Me More ›

How long does an Eureka math lesson take? ›

Not all Eureka Math lessons are formatted in the same way, but lessons in the same grade-band all follow a similar structure. Lessons in A Story of Units (PK-5) are written for a 60-minute class period, except for Pre-K lessons, which are 25 minutes, and K lessons, which are 50 minutes*.

Learn More Now ›

What are the 4 parts of the TEKS? ›

Explore how the TEKS are organized by Introduction, Knowledge and Skill Statement, Strand, and Student Expectations across a grade level or course. Recognize and differentiate between cognitive and content expectations noted in the TEKS.

Is Eureka math the same as common core? ›

Eureka Math, a Common Core-aligned curriculum published by the non-profit Great Minds Inc., equates mathematical concepts to stories, with the aim of developing conceptual understanding.

Know More ›

Is Eureka Math scripted? ›

The writers of the Eureka Math® curriculum deliberately designed lessons in the style of vignettes that illustrate what interaction between teacher and student might sound like in a classroom that is rolling out a rigorous curriculum.

Keep Reading ›

Who is the father of math Eureka? ›

Here's a closer look into this sudden discovery (the “Eureka!” moment): The famous Greek mathematician, physicist, and astronomer, Archimedes was born in 287 BC in Syracuse, a Greek colony in Sicily (an island now part of Italy).

Learn More Now ›

Who created Eureka math? ›

Together, these two math resources are the most commonly used in US schools. Working with educators and experts, Great Minds PBC has also developed Eureka Math ²™, Wit & Wisdom^® ELA, Geodes^® books for emerging readers, and PhD Science^®.

Discover More ›

What is the highest level of math in 9th grade? ›

9th grade math usually focuses on Algebra I, but can include other advanced mathematics such as Geometry, Algebra II, Pre-Calculus or Trigonometry.

Discover More ›

What is the hardest math in 5th grade? ›

Some of the hardest math problems for fifth graders involve multiplying: multiplying using square models, multiplying fractions and whole numbers using expanded form, and multiplying fractions using number lines.

Show Me More ›

What is advanced math in 8th grade called? ›

Almost every school district in the state offers an accelerated math option for selected students. These students take Algebra I in 8th grade. These students complete Algebra II, Geometry and Precalculus one year earlier than their peers. This allows them to take AP Calculus A/B in their senior year.

Keep Reading ›

What grade level is go math for? ›

Go Math! (K-6) on Ed is an easy-to-implement core curriculum with an effective instructional approach that includes robust differentiation and assessment resources that engage all levels of learners and support all levels of teachers, from novice to master.

Learn More ›